Blog Details

To calculate the thickness of a drum for a hoist crane, we need to consider several factors such as the working load, material properties, internal pressure (if any), and safety standards. The calculation typically involves principles of mechanical design and strength of materials.

Here’s a step-by-step approach:

. Known Parameters

• Diameter of the drum (D): Outer diameter of the drum.
• Material properties:
  • Yield strength (Sy​) of the material.
  • Allowable stress (Sa​) based on safety factor.
• Internal pressure (P): If the drum is subjected to internal pressure, this needs to be considered.
• Hoisting load (F): The maximum load the drum will handle.
• Safety factor (SF): Typically between 4 and 6 for cranes.
• Drum wall thickness (t): This is what we are solving for.

2. Assumptions

• The drum is cylindrical with uniform thickness.
• The material is homogeneous and isotropic.
• The drum is designed to withstand both external loads (hoisting force) and internal pressure (if applicable).

3. Calculation Methods

Case 1: Drum Subjected Only to Hoisting Load

If the drum is not subjected to significant internal pressure, the primary concern is the bending stress caused by the hoisting load. The formula for the required thickness can be derived from the bending stress equation:

Where:

• $\sigma$ = Stress in the material
• $M$ = Bending moment
• $c$ = Distance from the neutral axis to the outer fiber (radius of the drum)
• $I$ = Moment of inertia of the cross-section

For a thin-walled cylinder:

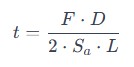
Where:

• $t$ = Thickness of the drum
• $F$ = Hoisting load
• $D$ = Diameter of the drum
• Sa​ = Allowable stress
• $L$ = Length of the drum

Where:

• σh​ = Hoop stress
• $P$ = Internal pressure
• $D$ = Diameter of the drum
• Sa​ = Allowable stress

Case 3: Combined Loading

If the drum is subjected to both hoisting load and internal pressure, the total stress must not exceed the allowable stress. In this case, the thickness must satisfy both conditions: